hypocycloidRose.gif
Take my revolution
hypocycloidRose.gif

Take my revolution
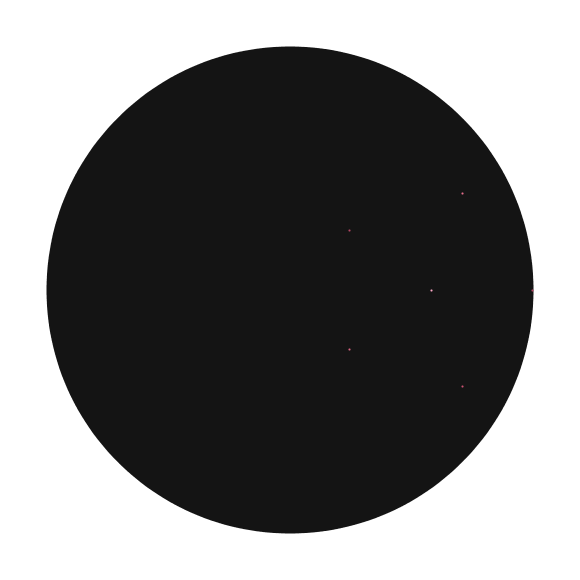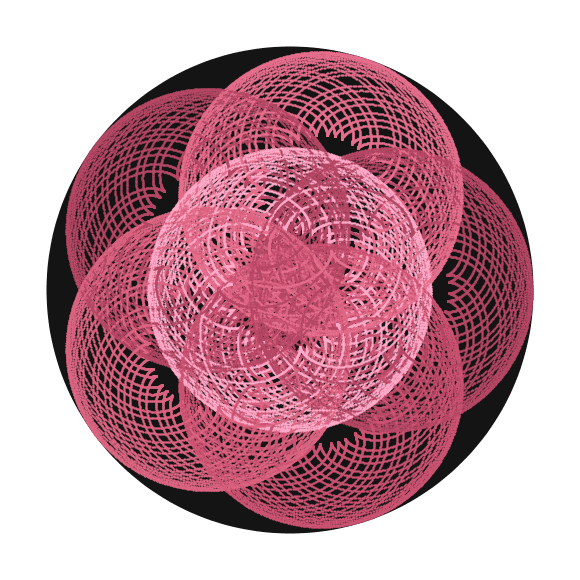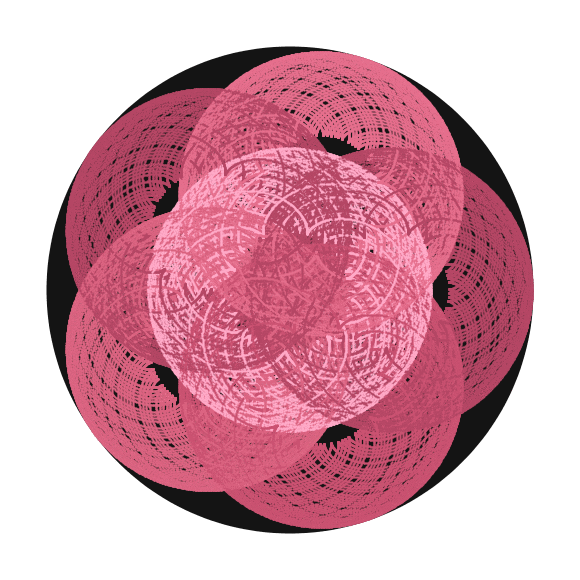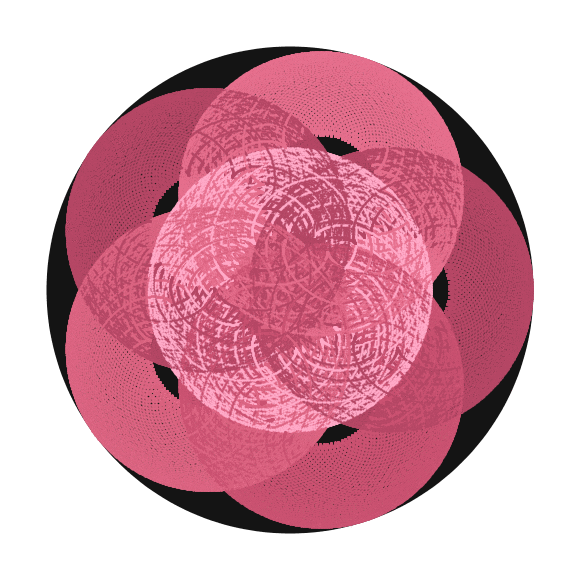
A hypocycloid is a curve traced by a point sitting on the circumference of one circle as that circle rolls around the inside of the circumference of another circle. The parametric equation for a hypocycloid is much like the parametric equation for a circle, although with a lot of extra doodads:
$$x = (a-b)cos(\theta) +b cos\left(\frac{a-b}{b}\theta\right)$$ $$y = (a-b)sin(\theta) +b sin\left(\frac{a-b}{b}\theta\right) $$where \(b\) is the radius of the inside, rolling circle and \(a\) is the radius of the outside circle.
So there are six overlapping hypocycloids here.
Code: Download hypocycloidRose.zip || Github